Abstract
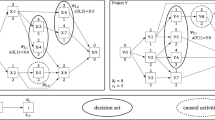
We model the working of a civil engineering firm concerned with land development as a three stage flexible flowshop with weak chain precedence constraints and where preemption is allowed. The scheduling objective is to minimize the total tardiness for all the projects. Since solving this problem optimally is very hard, we propose a number of heuristic scheduling procedures which are evaluated extensively on real-life data and artificial problem instances.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
Explore related subjects
Discover the latest articles and news from researchers in related subjects, suggested using machine learning.References
Blazewicz, J., Eiselt, H., Finke, G., Laporte, G., and Weglarz, J. (1991). “Scheduling jobs and vehicles in flexible manufacturing systems”, The International Journal of FMS, 4, 5–16.
Blazewicz, J., Ecker, K., Schmidt, G., and Weglarz, J., (1993). Scheduling in Computer and Manufacturing Systems. Springer-Verlag, Berlin 1993.
Blazewicz, J., Dror, M., Pawlak, G., and Stecke, K.E., (1994). “A note on flexible flowshop scheduling with two-stages”, Foundation of Computing and Decision Science 19, 159–172.
Dell'Olmo, P., Dror, M., and Kubiak, W., (1993). “‘Strong’-‘Weak’ Chain Constrained Scheduling.” working paper. MIS dept., University of Arizona.
Emmons, H. (1969). “One-machine sequencing to minimize certain functions of job tardiness. Operations Research, 17, 701–715.
Garey, M.R., Johnson, D.S., and Sethi, R., (1976). “The complexity of flowshop and jobhop scheduling”, Mathematics of Operations Research, 117–129.
Hefetz, N. and Adiri, I., (1982). “A note on the influence of missing operations on scheduling problems”, Naval Research Logistics Quarterly 29, 535–539.
Jackson, J.R. (1955). “Scheduling a production line to minimize maximum tardiness”, Research Report 43, Management Science Research Project, University of California, Los Angeles.
Kouvelis, P. and Vairaktarakis, G.L., (1994). “A two stage flexible job shop scheduling problem”, Operations Research Letters, (in press).
Law, Averill M. and Kelton, David W. (1991). “Simulation Modeling & Analysis”, cond Edition, McGraw-Hill, Inc. New York.
Sriskandarajah, C. and Sethi, S.P., (1989). “Scheduling algorithms for flexible flowshops: Worst and average case performance”, EJOR. 43, 143–160.
Wittrock, R.J., (1988). “An adaptable scheduling algorithm for flexible flow lines”, Operations Research, 36, 445–453.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Dror, M., Mullaseril, P.A. Three stage generalized flowshop: Scheduling civil engineering projects. J Glob Optim 9, 321–344 (1996). https://doi.org/10.1007/BF00121677
Accepted:
Issue Date:
DOI: https://doi.org/10.1007/BF00121677