Abstract
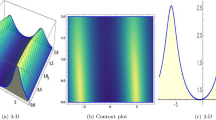
The main purpose of this article is to analyze the bifurcation, chaotic behaviors, and solitary wave solutions of the fractional Twin-Core couplers with Kerr law non-linearity by using the planar dynamical system method. This equation has profound physical significance and application value in the areas of optics and optical communication. Firstly, the traveling wave transformation is applied to convert the beta-derivative Twin-Core couplers with Kerr law non-linearity into the ordinary differential equations. Secondly, phase portraits and Poincaré sections of two-dimensional dynamical system and its perturbation system are plotted by using mathematical software. For different initial values, the planar phase diagram and three-dimensional phase diagram in red and blue are plotted, respectively. Finally, the solitary wave solutions of the fractional Twin-Core couplers with Kerr law non-linearity are obtained by using theory of planar dynamical system. In addition, three-dimensional graphs, two-dimensional graphs, and the contour graphs of the solitary wave solutions are drawn.
Similar content being viewed by others
Introduction
Dynamic systems1,2,3,4,5,6,7,8,9,10,11,12,13 are typically used to describe the evolution of a point in geometric space over time. It is a complex interdisciplinary theoretical system that involves multiple fields such as mathematics, physics, transportation, aerospace, and engineering technology. The study of dynamic system theory provides important mathematical models and solutions for solving many practical problems. The study of dynamical systems originated from the study of ordinary differential equations(ODEs). As early as 1881, French mathematician Paincaré founded the study of qualitative theory of ODEs, and introduced the concepts of bifurcation theory and singularities. He introduced the concept of phase space and represented the solution of ODEs as curves in phase space without solving ODEs. Thus, the properties of solutions to ODEs can be studied intuitively. In 1892, Russian mathematician Lyapunov systematically studied the stability of differential equations in his doctoral thesis, and proposed the famous Lyapunov stability theory14. The main characteristic of the qualitative theory of differential equations and Lyapunov stability theory mentioned above is that without solving the equations. After more than a century of rapid development, the qualitative theoretical theory of differential equations has penetrated into many important disciplines. In fact, when the parameters of the system change and pass a certain critical value, the global behavior of the system will suddenly change, which is usually referred to as bifurcation phenomenon. In nature, bifurcation is a common natural phenomenon. In recent years, with the rapid development of science and technology, the theory of dynamic system has become a very powerful mathematical tool. In recent years, Professor Li15,16,,16 has proposed the “three-step method” to study the branches and dynamic properties of traveling wave solutions for nonlinear partial differential equations (NLPDEs)17,18,19 by using qualitative theory of dynamical systems, and the traveling wave solution of NLPDEs are constructed. On the one hand, Professor Li Jibin’s “three-step method” provides a very important solution method for solving traveling wave solutions20,21,22,23,24 of NLPDEs, Through this method, many traveling wave solutions of NLPDEs can be obtained. On the other hand, dynamic system analysis theory can be used to obtain some dynamic characteristics of NPLDEs without solving NLPDEs. However, research on the dynamic behavior of NLPDEs with fractional derivatives25 and the construction of traveling wave solutions are still in their early stages. In this paper, the main purpose of this article is to analyze the bifurcation, chaotic behaviors, and solitary wave solutions of the fractional Twin-Core couplers with Kerr law non-linearity by using the planar dynamical system method.
The fractional Twin-Core couplers with Kerr law non-linearity is described as follows26
where \(u=u(x,t)\) and \(v=v(x,t)\) represent the optical problems in two cores, which are the complex-valued functions. \(^{A}_{0}D_{t}^{\beta }\) and \(^{A}_{0}D_{x}^{\beta }\) are the Atangana’s derivatives about the time and space. \(\alpha _{1}\), \(\alpha _{2}\), \(g_{1}\) and \(g_{2}\) stand for the coefficients of the dispersion terms. \(l_{1}\), \(l_{2}\), \(m_{1}\) and \(m_{2}\) are the constants. When \(\beta =1\), Eqs. (1) become an integer-order Twin-Core couplers model27. The Twin-Core couplers has important physical significance and application value in the fields of optics and optical communication. These couplers are typically composed of two parallel and close optical waveguides or “cores” that exchange energy through optical coupling.
The remaining part of this article is arranged as follows: In “Bifurcation and chaotic behaviors” section, the phase portraits and Poincaré sections of two-dimensional dynamical system and its perturbation system are discussed. In “Solitary wave solutions of Eqs. (1)” section, the solitary wave solutions of the fractional Twin-Core couplers with Kerr law non-linearity are constructed. In “Conclusion” section, a brief conclusion is given.
Bifurcation and chaotic behaviors
Preliminary
Definition 2.1
(Atangana’s fractional derivative)28 For \(\beta \in (0,1]\), the Atangana’s fractional derivative of \(f:[0,+\infty )\rightarrow (-\infty ,+\infty )\) is defined as
The Atangana’s fractional derivative is also known as the beta fractional derivative, which plays a very important role in the study of exact solutions to fractional partial differential equations. In recent years, there have been many literature reports on this work, and its definition, properties and applications can be referred to in the literature29,30,31,32,33.
Mathematical derivation
Firstly, let us the the wave transformation
where \(U(\xi )\) and \(V(\xi )\) are the wave’s amplitude component. c, \(\psi (x,t)\), \(\omega\), \(\kappa\), and \(\theta _{0}\) represent the frequency, the phase component, the wave number and the phase constant, respectively.
Substituting Eq. (2) into Eqs. (1), we obtain the imaginary component
From Eq. (3), we have
From Eq. (4), we known that the soliton speed should be equal. So we have
Similarly, when substituting Eq. (2) into Eqs. (1), we can also obtain the real part
Due to the balancing rectitude, we assume that \(U_{j}=U_{j^{*}}\). When \(F(h)=sh\), Eq. (6) can be transformed into
Here, Eq. (7) is rewritten as
when \(l_{j}=g_{j}=0\).
Qualitative analysis
When \(\alpha l\ne 0\), two-dimensional dynamic system of Eq. (8) can be rewritten as
with Hamiltonian function
where \(\Im _{3j}=\frac{\alpha _{j}\omega ^{2}+\kappa +k_{j}}{\alpha l^{2}}\) and \(\Im _{1j}=\frac{s_{j}}{\alpha l^{2}}\).
Assume that \(G(U_{j})=\Im _{3j}U_{j}^{3}-\Im _{1j}U_{j}\) is the abscissa of the equilibrium point. Further suppose that \(\textbf{M}(U_{j},0)=\left( \begin{matrix} 0 & 1\\ 3\Im _{3j}U_{j}^{2}-\Im _{1j}& 0 \end{matrix}\right)\) is the coefficient matrix of (9) at the equilibrium point. Then, we obtain
Based on the theory of planar dynamical systems, we can draw the following conclusions:
If \(\Im _{1}\Im _{3}<0\), the system (9) has one equilibrium point (0, 0).
If \(\Im _{1}\Im _{3}>0\), the system (9) has three equilibrium point (0, 0), \(\left( \sqrt{\frac{\Im _{1j}}{\Im _{3j}}},0\right)\), \(\left( -\sqrt{\frac{\Im _{1j}}{\Im _{3j}}},0\right)\).
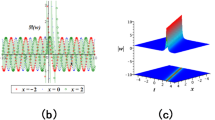
Due to the fact that Eqs.(1) is a coupled equation. Therefore, system (9) would also be two possibilities. When \(j=1\), we draw the planar phase portrait of system (9) as shown in Fig. 1.
2D phase portrait of system (9). (a) \(\Im _{11} < 0, \Im _{31} > 0\) (b) \(\Im _{11} > 0, \Im _{31} < 0\) (c) \(\Im _{11} < 0, \Im _{31} < 0\) (d) \(\Im _{11} > 0, \Im _{31} > 0\)
Qualitative analysis with perturbation term
In this section, two small perturbation term to the system (9) are added as follows
where \(f_{j}(\xi )=A_{j}\sin (\varpi \xi )\) represents the periodic disturbance term. \(f_{j}(\xi )=A_{j}e^{-0.07\xi }\) stands for the small disturbance. \(A_{j}\) and \(\varpi\) represent the amplitude and the frequency of system (12), respectively. By giving the parameters \(\Im _{11},\Im _{31},A,\varpi\), the two-dimensional, three-dimensional phase portrait, and Paincaré section diagrams of system (12) are drawn as shown in Figs. 2, 3, 4, and 5. Moreover, the branch phase diagram of system (12) are plotted as shown in Figs. 6 and 7.
The chaotic behaviors of system (12) with \(\Im _{11}=1,\Im _{31}=-5,A=1.6,\varpi =0.8\). (a) 2D phase portrait (b) 3D phase portrait (c) Paincaré section.
The chaotic behaviors of system (12) with \(\Im _{1}=1,\Im _{31}=-5,A=1.6\). (a) 2D phase portrait (b) 3D phase portrait (c) Paincaré section.
The chaotic behaviors of system (12) with \(\Im _{11}=-1,\Im _{31}=-5,A=1.6,\varpi =1.6\). (a) 2D phase portrait (b) 3D phase portrait (c) Paincaré section.
The chaotic behaviors of system (12) with \(\Im _{11}=-1,\Im _{31}=-5,A=1.6\). (a) 2D phase portrait (b) 3D phase portrait (c) Paincaré section.
The bifurcation portraits of system (12) with \(\Im _{11}=-1,\Im _{31}=-1\), \(r=\sqrt{z_1^{2}+U_1^{2}}\). (a) \(\varpi = 0.8\) (b) \(\varpi = 1\) (c) \(\varpi = 1.2\).
The bifurcation portraits of system (12) with \(\Im _{11}=1,\Im _{31}=-1\), \(r=\sqrt{z_1^{2}+U_1^{2}}\). (a) \(\varpi = 0.8\) (b) \(\varpi = 1\) (c) \(\varpi = 1.2\).
Solitary wave solutions of Eqs. (1)
In this section, we first assume that \(h_{0}=H(0,0)=0\), \(h_{1}=H(\pm \sqrt{\frac{\Im _{1j}}{\Im _{3j}}},0)=\frac{\Im _{1j}^{2}}{4\Im _{3j}}\). According to the analysis theory of planar dynamical systems, we can provide the solitary wave solutions of Eqs. (1).
\(\Im _{1j}>0,\) \(\Im _{3j}>0,\) \(0<h<\frac{\Im _{1j}^{2}}{4\Im _{3j}}\)
Then system (9) is rewritten as
where \(\vartheta _{1h}^{2}=\frac{\Im _{1j}+\sqrt{\Im _{1j}^{2}-4\Im _{3j}h}}{\Im _{3j}}\) and \(\vartheta _{2h}^{2}=\frac{\Im _{1j}-\sqrt{\Im _{1j}^{2}-4\Im _{3j}h}}{\Im _{3j}}\).
Plugging (13) into \(\frac{dU_{j}}{d\xi }=z_{j}\) and integrating it, the Jacobian function solutions are given
\(\Im _{1j}>0,\) \(\Im _{3j}>0,\) \(h=\frac{\Im _{1j}^{2}}{4\Im _{3j}}\)
When \(\vartheta _{1h}^{2}=\vartheta _{2h}^{2}=\frac{\Im _{1j}}{\Im _{3j}}\), the solitary wave of Eqs. (1) are given
\(\Im _{1j}<0,\) \(\Im _{3j}<0,\) \(\frac{\Im _{1j}^{2}}{4\Im _{3j}}<h<0\)
Then system (9) is rewritten as
where \(\vartheta _{3h}^{2}=\frac{\Im _{1j}-\sqrt{\Im _{1j}^{2}-4\Im _{3j}h}}{\Im _{3j}}\) and \(\vartheta _{4h}^{2}=\frac{\Im _{1j}+\sqrt{\Im _{1j}^{2}-4\Im _{3j}h}}{\Im _{3j}}\).
Plugging (14) into \(\frac{dU_{j}}{d\xi }=z_{j}\) and integrating it, the Jacobian function solutions are given
\(\Im _{1j}<0,\) \(\Im _{3j}<0,\) \(h=0\)
When \(\vartheta _{3h}^{2}=\frac{2\Im _{1j}}{\Im _{3j}},\vartheta _{4h}^{2}=0\), the solitary wave of Eqs. (1) are given
\(\Im _{1j}<0,\) \(\Im _{3j}<0,\) \(h>0\)
Then system (9) is rewritten as
where \(\vartheta _{5h}^{2}=\frac{\Im _{1j}+\sqrt{\Im _{1j}^{2}-4\Im _{3j}h}}{\Im _{3j}}\) and \(\vartheta _{6h}^{2}=\frac{\Im _{1j}-\sqrt{\Im _{1j}^{2}-4\Im _{3j}h}}{\Im _{3j}}\).
Plugging (15) into \(\frac{dU_j}{d\xi }=z_{j}\) and integrating it, the Jacobian function solutions are given
Numerical simulations
In this section, we plotted the solutions \(u_{1}(x,t)\) and \(u_{2}(x,t)\) including three-dimensional graph, two-dimensional graph, density graph, and counter graph as shown in Figs. 8 and 9.
Conclusion
In the article, we study the bifurcation, the chaotic behaviors and the solitary wave solutions for Eqs. (1) in the areas of optics and optical communication by using the theory of dynamical system, we draw the planar phase diagram of two-dimensional dynamical system. In addition, we draw plane phase diagrams, three-dimensional phase diagrams, and Paincaré section of the perturbed systems by adding periodic disturbances and small disturbances, respectively. For different initial values, we draw the planar phase diagram and three-dimensional phase diagram in red and blue, respectively. Based on the theory of planar dynamic system analysis, we obtain the solution to Eqs. (1). By using mathematical software, the three-dimensional, two-dimensional, density, and contour maps of these solutions are displayed. Compared with existing literature26, we not only obtained the dynamic behavior of system (9) and its perturbed system, but also obtained the Jacobian function solution of system (1) under specific parameter conditions. These have not been reported in other literature. The dynamic behavior and solitary wave solutions of fractional order nonlinear partial differential equations are currently hot topics in research, and in future studies, we will continue to focus on research in this area. Moreover, we will consider more complex fractional order nonlinear partial differential equations.
Data availability
The datasets used and/or analyzed during the current study available from the corresponding author on reasonable request.
References
Ali, K. K., Faridi, W. A. & Tarla, S. Phase trajectories and chaos theory for dynamical demonstration and explicit propagating wave formation. Chaos Soliton Fract.182, 114766 (2024).
Jhangeer, A., Faridi, W. A. & Alshehri, M. The study of phase portraits, multistability visualization, Lyapunov exponents and chaos identification of coupled nonlinear volatility and option pricing model. Eur. Phys. J. Plus139, 658 (2024).
Ali, K. K. et al. Bifurcation analysis, chaotic structures and wave propagation for nonlinear system arising in oceanography. Results Phys.57, 107336 (2024).
Murad, M. A. et al. Two distinct algorithms for conformable time-fractional nonlinear Schrödinger equations with Kudryashov’s generalized non-local nonlinearity and arbitrary refractive index. Opt. Quantum Electron.56, 1320 (2024).
Faridi, W. A. et al. Analyzing optical soliton solutions in Kairat-X equation via new auxiliary equation method. Opt. Quantum Electron.56, 1317 (2024).
Faridi, W. A. et al. The Lie point symmetry criteria and formation of exact analytical solutions for Kairat-II equation: Paul-Painlevé approach. Chaos Soliton Fract.182, 114745 (2024).
Liu, C. Y. The chaotic behavior and traveling wave solutions of the conformable extended Korteweg-de-Vries model. Open Phys.22, 20240069 (2024).
Laakmann, F. & Boullé, N. Bifurcation analysis of a two-dimensional magnetic Rayleigh-Bénard problem. Phys. D.467, 134270 (2024).
Tang, C., Li, X. Q. & Wang, Q. Mean-field stochastic linear quadratic optimal control for jump-diffusion systems with hybrid disturbances. Symmetry16, 642 (2024).
Fan, H. G. et al. Time-varying function matrix projection synchronization of Caputo fractional-order uncertain memristive neural networks with multiple delays via mixed open loop feedback control and impulsive control. Fractal Fract.8, 301 (2024).
Wang, J. & Li, Z. A dynamical analysis and new traveling wave solution of the fractional coupled Konopelchenko–Dubrovsky model. Fractal Fract.8, 341 (2024).
Tan, Z. Z., Hu, R. & Fang, Y. P. A second order dynamical system method for solving a maximally comonotone inclusion problem. Commun. Nonlinear Sci.134, 108010 (2024).
Everett, S. On the use of dynamical systems in cryptography. Chaos Soliton Fractals183, 1144952 (2024).
Xu, X. et al. Generalized Lyapunov stability theory of continuous-time and discrete-time nonlinear distributed-order systems and its application to boundedness and attractiveness for networks models. Commun. Nonlinear Sci.134, 108010 (2024).
Wu, J. & Yang, Z. Global existence and boundedness of chemotaxis-fluid equations to the coupled Solow-Swan model. AIMS Math.128, 107664 (2023).
Li, Z. & Liu, C. Y. Chaotic pattern and traveling wave solution of the perturbed stochastic nonlinear Schrödinger equation with generalized anti-cubic law nonlinearity and spatio-temporal dispersion. Results Phys.56, 107305 (2024).
Wu, J. & Huang, Y. J. Boundedness of solutions for an attraction-repulsion model with indirect signal production. Mathematics12, 1143 (2024).
Li, J. B., Han, M. A. & Ke, A. Bifurcations and exact traveling wave solutions of the Khorbatly’s geophysical Boussinesq system. J. Math. Anal. Appl.537, 128263 (2024).
Li, J. B. & Shi, J. P. Bifurcations and exact solutions of ac-driven complex Ginzburg–Landau equation. Appl. Math. Comput.221, 102–110 (2013).
Liu, C. Y. & Li, Z. The dynamical behavior analysis and the traveling wave solutions of the stochastic Sasa–Satsuma Equation. Qual. Theor. Dyn. Syst.23, 157 (2024).
Li, Z. & Hussain, E. Qualitative analysis and optical solitons for the (1+1)-dimensional Biswas-Milovic equation with parabolic law and nonlocal nonlinearity. Results Phys.56, 107304 (2024).
Gu, M. S., Peng, C. & Li, Z. Traveling wave solution of (3+1)-dimensional negative-order KdV-Calogero-Bogoyavlenskii-Schiff equation. AIMS Math.9, 6699–6708 (2024).
Tang, Y. & Rezazadeh, H. On logarithmic transformation-based approaches for retrieving traveling wave solutions in nonlinear optics. Results Phys.51, 106672 (2023).
Khater, M. M. A. Computational simulations of propagation of a tsunami wave across the ocean. Chaos Soliton Fractals174, 113806 (2023).
Tang, L. Dynamical behavior and multiple optical solitons for the fractional Ginzburg-Landau equation with \(\beta\)-derivative in optical fibers. Opt. Quantum Electron.56, 175 (2024).
Asad, A., Riaz, M. B. & Geng, Y. F. Sensitive demonstration of the Twin-Core couplers including Kerr law non-linearity via beta derivative evolution. Fractal Fract.6, 697 (2022).
Arnous, A. et al. Solitons in nonlinear directional couplers with optical metamaterials by trial function scheme. Acta Phys. Pol. A132, 1399–1410 (2017).
Li, Z. Bifurcation and traveling wave solution to fractional Biswas-Arshed equation with the beta time derivative. Chaos Soliton Fractals160, 112249 (2022).
Akrami, M. H. & Owolabi, K. M. On the solution of fractional differential equations using Atangana’s beta derivative and its applications in chaotic systems. Sci. Afr.21, e01879 (2023).
Atangana, A., Baleanu, D. & Alsaedi, A. Analysis of time-fractional Hunter-Saxton equation: A model of neumatic liquid crystal. Open Phys.14, 145–149 (2016).
Iqbal, M. A., Akbar, M. A. & Islam, M. A. The nonlinear wave dynamics of fractional foam drainage and Boussinesq equations with Atangana’s beta derivative through analytical solutions. Results Phys.56, 107251 (2024).
Li, Z. & Peng, C. Bifurcation, phase portrait and traveling wave solution of time-fractional thin-film ferroelectric material equation with beta fractional derivative. Phys. Lett. A484, 129080 (2023).
Chakrabarty, A. K. et al. Dynamical analysis of optical soliton solutions for CGL equation with Kerr law nonlinearity in classical, truncated M-fractional derivative, beta fractional derivative, and conformable fractional derivative types. Results Phys.60, 107636 (2024).
Funding
This research was funded by Key Laboratory of Numerical Simulation of Sichuan Provincial Universities(Grant. 2023SZFZ003) and the Science and Technology Department of Sichuan Province via the grant (Grant no. 2023NSFSC1344).
Author information
Authors and Affiliations
Contributions
All authors reviewed the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License, which permits any non-commercial use, sharing, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if you modified the licensed material. You do not have permission under this licence to share adapted material derived from this article or parts of it. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by-nc-nd/4.0/.
About this article
Cite this article
Li, Z., Lyu, J. & Hussain, E. Bifurcation, chaotic behaviors and solitary wave solutions for the fractional Twin-Core couplers with Kerr law non-linearity. Sci Rep 14, 22616 (2024). https://doi.org/10.1038/s41598-024-74044-w
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-024-74044-w
Keywords
This article is cited by
-
Optical solitons, bifurcation, and chaos in the nonlinear conformable Schrödinger equation with group velocity dispersion coefficients and second-order spatiotemporal terms
Scientific Reports (2025)
-
Exploring the chaotic, sensitivity and wave patterns to the dual-mode resonant Schrödinger equation: application in optical engineering
Scientific Reports (2025)
-
Bifurcation, chaos, modulation instability, and soliton analysis of the schrödinger equation with cubic nonlinearity
Scientific Reports (2025)
-
Optical solutions to the time fractional Akbota equation arising in nonlinear optics via two distinct methods
Scientific Reports (2025)
-
Study of a generalized stochastic scale-invariant analogue of the Korteweg-de Vries equation
Nonlinear Dynamics (2025)