Introduction

China is the world’s largest producer and consumer of vegetables, and the planting area of vegetables also ranks first in the world1. And heading vegetables is the largest proportion of vegetables in China’s planting and consumption of a category, including kale, cabbage, heading lettuce and many other subdivided vegetable varieties. However, China’s heading vegetable mechanized harvesting level is low, still completely rely on manual completion. During harvesting process, farmers need to constantly bend down and use knives to cut vegetables from the rhizome, and then remove the exclusion of wrapped leaves, picking up the vegetable bulb, and finally loaded and transported and so on. Therefore the harvesting operation time is long, and the labor intensity is great. The mechanized harvesting of cabbage-type vegetables can significantly improve harvesting efficiency, reduce labor costs, and promote sustainable agricultural production. However, due to uneven growth, complex structures, and poor equipment adaptability, mechanized harvesting faces high technical challenges and reliability issues, requiring further technological innovation and optimization.

Tong et al. designed a hand-held kale harvester, based on the mechanical properties of kale and Ansys modal analysis of the key components of the harvester design verification, and trial production prototype for field testing, the prototype harvesting yield of 92.8%, the effective cutting rate of 93.5%, machine picking damage rate of 6.6%, the machine picking loss rate of 4.2%, the performance indexes performed well, providing a reference for kale harvesting and structural improvement2. Kim et al. designed a tracked self-propelled cabbage harvester and optimized the pulling device of the machine. Field tests showed that the pulling effect was better when the inclination angle of the pulling device was 30°, the walking speed of the harvester was 0.4 m/s, and the clamping and conveying speed was 0.61 m/s3. However, because the pulling mechanism was optimized for the harvesting of cabbage, it was not possible to optimize the optimization of the pulling device. Du et al. designed a self-propelled kale harvester and carried out field tests for the prototype, the prototype harvesting speed of 0.3 m/s, the success rate of pulling 86.7%, conveying the success rate of 93.3%, cutting the roots of the qualified rate of 75.0%, peeling the leaves of the qualified rate of 81.7%, basically to meet the requirements of the kale mechanized harvesting4.

At this stage, domestic and foreign research on heading vegetables harvesting technology is mainly focused on the coordination of the key functional components of the research field. There is a relative lack of research on the physical and mechanical properties of heading vegetables and low damage to cut roots5. Aiming at the importance of the root cutting process in the mechanized harvesting process of heading vegetables, a common root cutting device for heading vegetables was designed, through the analysis of the movement and force of the root cutting process of the roots of heading vegetables, the type of cutter combination of the root cutting device and the range of values of key parameters6. ANSYS software was used to carry out modal analysis of the blade and disk to verify the structural reasonableness of the designed cutter, and LS-DYNA was used to analyze the influence of different root-cutting parameters on the maximum root-cutting force and root-cutting internal energy7. After determining the parameters that have the greatest influence on the maximum root cutting force and internal energy of root cutting, the key parameters are optimized through orthogonal bench experiments to provide a theoretical basis for the structural improvement of root cutting device.

Root cutting device dynamic model of the cutting process and experimental design

Motion and force analysis of root cutting process

Root cutting is the most critical step in the harvesting process when heading vegetables are harvested. In order to ensure the performance of the root cutting device, accurate motion and force analysis of the root cutting process is required. When cutting roots, the motion of the disk cutter is compounded by the circular motion rotating around the root cutting axis and the linear motion advancing with the harvester. The root cutting process is shown as Fig. 1. In the actual root cutting process, the force in Z-axis is far smaller than X- axis and Y-axis. Because the root cutting process occur at XY-plane.

Fig. 1
figure 1

The process of root cutting.

Full size image

In order to analyze the trajectory of the knife edge of the disc cutter, it is simplified as shown in Fig. 2. Point A is the root point of the knife edge. Point B is the apex of the knife edge. point C is the root point of the adjacent knife edge. And point D as the apex of the adjacent knife edge.The X-Y coordinate system established is shown in Fig. 2. The Z-axis is perpendicular to the paper surface outwards. In the disc cutter blade selected A, B, C, D four points, the point of the equation of motion as shown in Eqs. (1), (2), (3), (4):

Fig. 2
figure 2

The trajectory of the knife edge of the disc cutter.

Full size image

The equation of the trajectory of point A on the blade:

$$\:\begin{array}{c}\left\{\begin{array}{c}{x}_{A}={v}_{m}t+r\text{cos}\theta\:\text{sin}\left(\omega\:t+{\upbeta\:}\right)\\\:{y}_{A}=r\text{cos}\left(\omega\:t+{\upbeta\:}\right)\\\:{z}_{A}=-r\text{sin}\theta\:\text{sin}\left(\omega\:t+{\upbeta\:}\right)\end{array}\right.\end{array}$$
(1)

The equation of the trajectory of point B on the blade:

$$\:\begin{array}{c}\left\{\begin{array}{c}{x}_{B}={v}_{m}t+R\text{cos}\theta\:\text{sin}\left(\omega\:t+\frac{{\upbeta\:}}{2}\right)\\\:{y}_{B}=R\text{cos}\left(\omega\:t+\frac{{\upbeta\:}}{2}\right)\\\:{z}_{B}=-R\text{sin}\theta\:\text{sin}\left(\omega\:t+\frac{{\upbeta\:}}{2}\right)\end{array}\right.\end{array}$$
(2)

The equation of the trajectory of point C on the blade:

$$\:\begin{array}{c}\left\{\begin{array}{c}{x}_{C}={v}_{m}t+r\text{cos}\theta\:\text{sin}\omega\:t\\\:{y}_{C}=r\text{cos}\omega\:t\\\:{z}_{C}=-r\text{sin}\theta\:\text{sin}\omega\:t\end{array}\right.\end{array}$$
(3)

The equation of the trajectory of point D on the blade:

$$\:\begin{array}{c}\left\{\begin{array}{c}{x}_{D}={v}_{m}t+R\text{cos}\theta\:\text{sin}\left(\omega\:t-\frac{{\upbeta\:}}{2}\right)\\\:{y}_{D}=R\text{cos}\left(\omega\:t-\frac{{\upbeta\:}}{2}\right)\\\:{z}_{D}=-R\text{sin}\theta\:\text{sin}\left(\omega\:t-\frac{{\upbeta\:}}{2}\right)\end{array}\right.\end{array}$$
(4)

where \(\:{v}_{m}\)—The forward speed of the harvester, \(\:mm/s\); \(\:t\)—The working time, s; \(\:\omega\:\)—The angular velocity of the disk cutter, \(\:rad/s\); \(\:R\)—The radius at the apex of the knife blade, \(\:mm\); \(\:r\)—The radius at the root point of the knife blade, \(\:mm\); \(\:\theta\:\)—The angle between the disk cutter and the horizontal plane, (°); \(\:{\upbeta\:}\)—The angle between two adjacent tooth blades, (°).

As shown in Fig. 2, when the blade vertex D moves for t2 time, it will arrive at the same longitudinal coordinate position (\(\:y=0\)) as the blade vertex B moves for t1 time. At this time \(\:\varDelta\:x\:\)is the distance between point C and point B. Through the blade trajectory equation, \(\:\varDelta\:x\) about the blade root point C movement time t2 and blade vertex B movement time t1 relationship can be got, as shown in Eq. (5).

$$\:\begin{array}{c}\left\{\begin{array}{c}\varDelta\:x={x}_{D}\left({t}_{2}\right)-{x}_{B}\left({t}_{1}\right)\\\:{y}_{B}\left({t}_{1}\right)=0\\\:{y}_{D}\left({t}_{2}\right)=0\end{array}\right.\end{array}$$
(5)

Solve by association(2), (4), (5) and get the equation of \(\:\varDelta\:x\):

$$\:\begin{array}{c}\varDelta\:x=\frac{\beta\:{v}_{m}}{\omega\:}\end{array}$$
(6)

In order to ensure that no cuts are missed during the root cutting process, it is necessary to ensure that the cutting pitch is greater than or equal to the height of the saw teeth, i.e., \(\:\varDelta\:x\le\:h=R-r\). The condition that no cuts are missed during the root cutting process can be obtained as:

$$\:\begin{array}{c}{v}_{m}\le\:\frac{\omega\:h}{\beta\:}\end{array}$$
(7)

where: \(\:h\)—The height of the saw teeth, mm.

The relationship between the angle between two adjacent tooth edges \(\:\beta\:\) and the number of the saw teeth:

$$\:\begin{array}{c}\beta\:Z=2\pi\:\end{array}$$
(8)

where: \(\:Z\)—The number of the saw teeth.

Solve by association (7), (8):

$$\:\begin{array}{c}{v}_{m}\le\:\frac{\omega\:hZ}{2\pi\:}=\frac{hnZ}{60}\end{array}$$
(9)

where: \(\:h\)—The height of the saw teeth, mm.

According to the design of this machine, take \(\:h=10\,mm\), \(\:Z=60\), the minimum working speed of the cutter \(\:{n}_{min}=100r/min\), the maximum forward speed of the machine can be calculated \(\:{v}_{mmax}=1\,m/s\), to meet the requirements of the forward speed of the harvester9.

After determining the non-leakage cutting conditions through motion analysis, it is also necessary to analyze the force on the root cutting process to determine the key parameters of the cutter. Since the blade is rotating when cutting roots, the teeth are in continuous contact with the roots. Therefore, the root cutting process can be simplified as a model of a double disk cutter cutting roots. In the root cutting process of heading vegetables, the disk cutter is mainly subject to the tangential force\(\:{\:F}_{t}\), radial force \(\:{F}_{n}\) and axial force \(\:{F}_{a}\), as shown in Fig. 3.

Fig. 3
figure 3

Force diagram of disc cutter.

Full size image

The radial force \(\:{F}_{n}\), tangential force \(\:{F}_{t}\) and axial force \(\:{F}_{a}\) are decomposed orthogonally and the force analysis is shown in Eq. (10):

$$\:\begin{array}{c}\left\{\begin{array}{c}{F}_{x0}={F}_{tx}-{F}_{nx}={F}_{t}\text{sin}\alpha\:-{F}_{n}\text{cos}\alpha\:\\\:{F}_{y0}={F}_{ty}+{F}_{ny}={F}_{t}\text{cos}\alpha\:+{F}_{n}\text{sin}\alpha\:\end{array}\right.\end{array}$$
(10)

Since Y0 and Y-axis are coincident in space, X0 and X-axis intersect at an angle \(\:\theta\:\) in space, the force on Y0-axis and Y-axis are equal, and the force on X0-axis has components on X-axis and Z-axis, the axial force \(\:{F}_{a}\) and the force on X0-axis \(\:{F}_{x0}\) are decomposed orthogonally, and the force is analyzed as shown in Eq. (11):

$$\:\begin{array}{c}\left\{\begin{array}{c}{F}_{x}={F}_{x0}\text{cos}\theta\:-{F}_{ax}=\left({F}_{t}\text{sin}\alpha\:-{F}_{n}\text{cos}\alpha\:\right)\text{cos}\theta\:-{F}_{a}\text{sin}\theta\:\\\:{F}_{y}={F}_{y0}={F}_{t}\text{cos}\alpha\:+{F}_{n}\text{sin}\alpha\:\\\:{F}_{z}={-F}_{x0}\text{sin}\theta\:-{F}_{az}=\left({F}_{n}\text{cos}\alpha\:-{F}_{t}\text{sin}\alpha\:\right)\text{sin}\theta\:-{F}_{a}\text{cos}\theta\:\end{array}\right.\end{array}$$
(11)

The magnitude of the combined cutting force on the disk cutter during root cutting is shown in Eq. (12):

$$\:\begin{array}{c}F=\sqrt{{F}_{x}^{2}+{F}_{y}^{2}+{F}_{z}^{2}}=\sqrt{{F}_{n}^{2}+{F}_{t}^{2}+{F}_{a}^{2}}\end{array}$$
(12)

This design of the root cutting device has overlapping upper and lower two disc knives. During the root cutting process, the double disk knives will clamp and cut off the root of the heading vegetable10. Take force analysis for the root cutting process of heading vegetable root, and the force diagram is shown in Fig. 4.

Fig. 4
figure 4

Stress analysis of heading vegetable root during root cutting process.

Full size image

The force analysis leads to Eq. (13):

$$\:\begin{array}{c}\left\{\begin{array}{c}{F}_{S}={F}_{Ty}+{F}_{Ny}={F}_{T}\text{sin}\alpha\:+{F}_{N}\text{cos}\alpha\:\\\:{F}_{C}={F}_{Tx}+{F}_{Nx}={F}_{T}\text{cos}\alpha\:-{F}_{N}\text{sin}\alpha\:\\\:{F}_{T}=f{F}_{N}\end{array}\right.\end{array}$$
(13)

where \(\:{F}_{N}\)—normal reaction force of disc cutter acting on heading vegetable roots, N; \(\:\alpha\:\)—angle between \(\:{F}_{N}\) and Y0 axis direction, (°); \(\:{F}_{T}\)—friction force of disk cutter on heading vegetable roots, N; \(\:f\)—friction coefficient between disk cutter and heading vegetable roots, generally taken as 0.4–0.7.

From the force analysis, the condition that the disk cutter can clamp the heading vegetable roots is: \(\:{F}_{T}\text{cos}\alpha\:>{F}_{N}\text{sin}\alpha\:\), therefore, when \(\:f>\text{tan}\alpha\:\), the disk cutter can clamp the heading vegetable roots.

$$\:\begin{array}{c}\alpha\:={\text{sin}}^{-1}\frac{\raisebox{1ex}{$A$}\!\left/\:\!\raisebox{-1ex}{$2$}\right.}{\raisebox{1ex}{$\left(D+d\right)$}\!\left/\:\!\raisebox{-1ex}{$2$}\right.}={\text{sin}}^{-1}\frac{A}{D+d}\end{array}$$
(14)

Where \(\:A\)—Center distance between two disc cutters, mm; \(\:D\)—Diameter of disk cutter, mm; \(\:d\)—Diameter of heading vegetable root cutting place, generally take 25–35 mm.

When \(\:A\) increases or \(\:D\) decreases, \(\:a\) decreases, which is conducive to the two disc cutters clamping the heading vegetable roots for cutting. Due to the two disc cutter center distance \(\:A\) and the disc cutter diameter \(\:D\) and other structural limitations, generally \(\:a\) take 35–40°. Due to the knotting of vegetable roots and the cutter edge friction coefficient f = 0.4. So the two light-edged disc cutter is difficult to clamp the knotting vegetable roots for cutting. It is necessary to change the shape of the cutting edge to improve the ability to clamp the heading vegetable roots. In this paper, the combination of a light-edged disk cutter and a serrated disk cutter is used to improve the ability of the cutter to clamp the roots.

Root cutting mechanism design

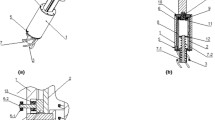
According to the analysis of the root cutting process of heading vegetables, the design of the root cutting device is shown in Fig. 5.

Fig. 5
figure 5

The root cutting mechanism. (1) Bracket, (2) Unidirectional coupling, (3) Hydraulic motor, (4) Cutting knife, (5) Knife plate, (6) Cutting shaft, (7) T-type commutator, (8) Hexagonal shaft.

Full size image

The root cutting mechanism is mainly composed of cutter, cutter disk, bracket, cutter shaft, universal joint coupling, T-type commutator, hexagonal shaft and hydraulic motor. Through the theoretical analysis, it is known that two disc light knife can’t hold the root well for cutting, so this paper chooses the combination of light knife + serrated knife to cut the root11.

Referring to the results of the previous analysis, in the case of the root cutting mechanism, conveying mechanism and the frame of the three do not interfere, while meeting the requirements of the two pieces of the knife has part of the overlap, the outer diameter of the knife is set to 270 mm, the diameter of the middle hole is set to 25 mm, the cutter parameter design is shown in Fig. 6.

Fig. 6
figure 6

The cutter parameter design.

Full size image

Materials and methods

The workpiece material and equipment

“Biyue Brand” cabbage was used in the experiment, which was collected in November 2024 from the cabbage planting field in Hanting District, Weifang City. The average height of this cabbage is 434.43 mm, the average diameter is 218.52 mm. At the time of collection, the cabbages were at the mature stage. Cabbage plants with comparable ball diameter, good growth, and free of pests and diseases were selected. The whole cabbage plant was pulled up from the ground. The root soil was removed as a test specimen, as shown at Fig. 712.The axial modulus of elasticity and radial modulus of elasticity were taken as 6.15Mpa and 4.36Mpa in the simulation tests.

Fig. 7
figure 7

Chinese cabbage plants for experiment.

Full size image

Root cutting test bench is mainly to complete the heading vegetables clamping transportation and root excision, its structure is shown in Fig. 8, mainly consists of double disc cutting mechanism, cutting motor, clamping conveying device, conveying motor, dynamic torque sensors, elastic sleeve pin coupling, frame, control and information acquisition system and other components. During the working process, the cabbage is stably clamped by the clamping and conveying device and conveyed in the direction of the cutting device, and the cabbage root is removed by the high-speed rotating double disc cutter at the bottom. The dynamic torque sensor in this test bench is connected to the power input and output shafts respectively through the elastic pin coupling at both ends. It is possible to collect the torque condition when the test bench is running. The dynamic toque sensors will collect the whole torque data and the idling torque. The root cutting power will be calculate by Eq. (15).

$$\:\text{P}=\frac{({\text{T}}_{1}-{\text{T}}_{0})\cdot\:\text{n}}{9550}$$
(15)
Fig. 8
figure 8

Design of Chinese cabbage root cutting test-bed. (1) Frame, (2) conveying motor, (3) clamping conveying device, (4) double disc cutting mechanism, (5) elastic sleeve pin coupling, (6) dynamic torque sensor, (7) cutting motor.

Full size image

Simulation model of cutter and cutter disk

According to the structure and parameters of the disc cutter blade designed in this paper, the diameter of the disc cutter blade is 270 mm, and the thickness is 2 mm.The 3D model of the disc cutter blade is established by SolidWorks 2020, and then the file is exported to the ANSYS finite element analysis software13. The properties of the blade and disk material are shown in Table 1.

Table 1 The properties of the blade and disk material.
Full size table

Then mesh division, add constraints, and mesh division of the disk blade. The disk blade is fixed with the disk by four bolts, and the four holes are fixed in the simulation model, and then the solution is started, and finally the intrinsic frequency and vibration pattern of the disk blade and the disk are viewed in the post-processing14.

Experimental design of factors affecting maximum root cutting force and internal energy of root cutting

In order to study the influence of different root-cutting parameters (rotational speed of the cutter, traveling speed, inclination angle of the cutter and overlap of the cutter) on the maximum root-cutting force and internal energy of the root-cutting, the range and level values of each factor is chosen as shown in Table 29. In the root-cutting numerical model, the corresponding factors were changed to the corresponding level values, and the other parameters were kept unchanged. LS-DYNA was used to simulate the numerical simulation of each group of experiments and record the data of the maximum root-cutting force and internal energy of root-cutting under different root-cutting parameters15.

Table 2 Simulation test factor level table.
Full size table

Orthogonal experimental design

According to the simulation experiments, the root cutting process in heading vegetable harvesting is affected by the rotational speed of the cutter, the inclination angle of the cutter, and the amount of overlap of the cutter. And in the root cutting process, the root cutting power is also affected by the conveying speed, so we chose to conduct a four-factor, three-level quadratic rotary orthogonal test with rotational speed of the cutter (A), conveying speed (B), inclination angle of the cutter (C) and overlap of the cutter (D) as the experimental factors, and root cutting power (R) as the response index, and the coding of the four factor levels of the root cutting test is shown in Table 3 .

Table 3 Experiments factor levels.
Full size table

Experimental results and discussion

Modal analysis of cutter blade and disk

Modal analysis of cutter blade

The results of the modal analysis of the disk cutter blade are shown in Table 4; Fig. 9.

Table 4 The first six natural frequencies of disk cutter blade.
Full size table
Fig. 9
figure 9

The first six natural frequencies of disk cutter blade.

Full size image

As can be seen from Table 4; Fig. 9, the 1st order vibration mode and 2nd order vibration mode of the disk cutter blade have the intrinsic frequencies of 187.86 Hz and 187.87 Hz, respectively, which are the vibration of local deformation of the front, back, left and right edges of the disk cutter blade; and the 3rd order vibration mode has the intrinsic frequency of 192.09 Hz, which is the vibration of axial deformation at the edge of the disk cutter blade; the intrinsic frequencies of the 4th and 5th order vibration patterns are 204.75 Hz and 249.31 Hz, respectively, for the vibration of the second-order twisted deformation of the disk cutter blade; and the intrinsic frequency of the 6th order vibration pattern is 386.89 Hz, for the vibration of the third-order twisted deformation of the disk cutter blade16.

The cutting speed of knotted vegetables is generally 100–500 rpm, which corresponds to a frequency much lower than the intrinsic frequency of the first 6 orders of vibration, avoiding the resonance phenomenon during the working process of the disk blade, proving the rationality of the structural design of the disk cutter blade17.

Modal analysis of the disk

The results of the modal analysis of the disk are shown in Table 5; Fig. 10.

Table 5 The first six natural frequencies of disk.
Full size table
Fig. 10
figure 10

The first six natural frequencies of disk.

Full size image

From Fig. 10; Table 5, it can be seen that the 1st order vibration mode and 2nd order vibration mode of the cutter plate have the intrinsic frequency of 580.22 Hz and 620.63 Hz respectively, which are the vibration of bending deformation of the cutter plate shaft at front and back, left and right. The intrinsic frequencies of the 3rd and 6th order vibration patterns are 1007.9 Hz and 1606.2 Hz, respectively, for the vibration of radial and axial deformation of the cutter disk; the intrinsic frequencies of the 4th and 5th order vibration patterns are 1458 Hz and 1487 Hz, respectively, for the vibration of front-back and left-right bending deformation of the cutter disk.

Through the above analysis, it can be seen that the corresponding resonance frequency of the cutter blade is much higher than the resonance frequency of the disc blade, indicating that it is difficult for the resonance phenomenon of the cutter blade to occur during the working process, which proves the reasonableness of the design of the cutter blade structure.

Analysis of the effect of different root-cutting parameters on maximum root-cutting force and internal energy of root-cutting

Through simulation experiments, the influence of maximum root-cutting force and internal energy of root-cutting under different root-cutting parameters were obtained as shown in Table 6 .

Table 6 Data of maximum root cutting force and internal energy under different root cutting parameters.
Full size table

From the simulation results, it can be seen that the maximum root cutting force decreases with the increase of the rotational speed of the cutter, and the maximum root cutting force is the largest at 100 r/min, which is 31.147 N. The maximum root cutting force decreased most significantly when the rotational speed of the cutter was between 100 r/min and 200 r/min. Because the rotational speed of the cutter increases, the sliding effect of the cutter on the cabbage root increases, and the root cutting force decreases. As the rotational speed of the cutter increases, the instability of the cutter increases, and the maximum root cutting force decreases less after the rotational speed of the cutter reaches 400 r/min. The internal energy of root cutting and the rotational speed of the cutter basically increased linearly, and the larger the rotational speed of the cutter, the higher the energy consumption of root cutting.

The maximum root cutting force increases with the increase of traveling speed, and the maximum root cutting force is the smallest at 100 r/min, which is 12.449 N. With the traveling speed between 0.2 m/s and 0.4 m/s, the maximum root cutting force has the most obvious upward trend. With the increase of the traveling speed, the area of the cutter cutting the roots per unit of time increases, the friction effect is strengthened, and the maximum root cutting force increases. As the traveling speed increases, the impact of the cutter on the root is more obvious. So after the traveling speed reaches 0.5 m/s, the maximum root cutting force is caused by the impact effect, and its change is small. The internal energy of root cutting decreases with the increase of traveling speed. When the other factor is determined, the cutting time becomes shorter as the travel time increased. And when the travel speed is not too large(less than 0.4 m/s), the impact effect is not apparent. Thus the cutting energy becomes lower. After the traveling speed reaches 0.4 m/s, the decreasing trend of the internal energy of root cutting slows down. Because the traveling speed is too large, the impact effect of the cutter on the roots increases, and the energy consumption of root cutting slows down.

Maximum root cutting force with the increase of the cutter inclination angle showed a trend of first decrease and then increase. In the cutter inclination angle of 10°, the maximum root cutting force is the smallest, 17.613 N. This can be the best root cutting inclination angle. When cutter tilt angle is in the range of 0–10°, the method of root cutting becomes cutting from cross cutting with the increase in the tilt angle of the cutter. And the cut force of cutting is far smaller than cross cutting19. Thus, the maximum root cutting force decreases. When the inclination angle of the cutter is more than 10°, with the increase of the inclination angle of the cutter, the clamping effect of the root of heading vegetables on the cutter is enhanced. The cut is not smooth, the impact of root cutting is increased, and the maximum root cutting force is increased. Cutting root internal energy with the increase in the inclination angle of the cutter was first reduced and then increased, this is due to the first period with the increase in the inclination angle of the cutter, the chipping effect is strengthened, the cutting root force is reduced, the cutting root energy consumption is reduced; the later period with the increase in the inclination angle of the cutter, heading vegetable roots on the cutter’s clamping effect is strengthened, the incision is not smooth, the cutting root energy consumption rises.

Maximum root cutting force with the increase of the knife overlap amount showed a trend of first decrease and then increase. In the knife overlap amount of 20 mm, the maximum root cutting force is the smallest, 20.499 N, this time for the best root cutting overlap amount. Cutting knife overlap in the range of 10–20 mm, with the increase of the overlap of the cutting knife, the root cutting point moves forward, the slip cutting angle increases, the cutting knife’s slip cutting effect is strengthened, and the maximum root cutting force decreases. In the range of 20–30 mm, the maximum root cutting force increased due to the increase in contact area between the cutter and heading vegetable roots and the increase in friction. The internal energy of root cutting showed a tendency of decreasing and then increasing with the increase of knife overlap, which was due to the fact that in the early stage, with the increase of knife overlap, the sliding cutting effect was strengthened, the root cutting force was reduced, and the energy consumption of root cutting was lowered; in the later stage, with the increase of contact area between the knife and heading vegetable roots, the friction effect was strengthened, and the energy consumption of root cutting was increased.

Analysis of orthogonal experiment results

The results of orthogonal experiments are shown in Table 7.

Table 7 The results of orthogonal experiments.
Full size table

A quadratic polynomial response surface regression model of the cut root power R on the cutter rotational speed A, conveying speed B, cutter inclination C, and cutter overlap D was established by using Design-Expert 8.06 to fit the multivariate regression to the experimental results in Table 7. The regression equation of the cut root power R is shown in Eq. (15).

$$\begin{aligned}R\,& =\,88.16\,+\,21.69\,A\,+\,13.53B - 1.09\,C - 0.15D\,+\,3.35AB\,+\,2.37AC\,+\,3.8AD\\ & \quad - 4.63BC\,+\,0.025BD\,+\,1.83CD - 3.38{A^2} - 1.71{B^2}\,+\,3.92{C^2}\,+\,0.17{D^2}\end{aligned}$$
(15)

The optimized regression fitting equation about the tangent root power obtained after removing the insignificant term is shown in Eq. (16), and the optimized model is reliable with P < 0.01 and the misfit term with P > 0.05.

$$R\,=\,87.61\,+\,21.69\,A\,+\,13.53B - 1.09\,C - 0.15D\,+\,3.35AB\,+\,3.8AD - 4.63BC - 3.22{A^2}\,+\,4.08{C^2}$$
(16)

Discussion

Response surface analysis was performed on the results of orthogonal experiments18, and in this paper, by fixing two of the factors at the zero level, we analyzed the relationship between the effects of the other two factors on the response index, tangent root power, and the response surface of their interactions is shown in Fig. 11.

Fig. 11
figure 11

Three-dimensional response surface analysis of root-cutting power interaction factors.

Full size image

In order to achieve low-power and high-efficiency root cutting, this paper takes the root cutting power as the optimization index, and carries out the parameter optimization analysis on the influencing factors such as the rotational speed of the cutter, the conveying speed, the inclination angle of the cutter, and the overlap of the cutter. The regression model is optimized and solved using the optimization module in Design Expert 8.0.6 software, and the established optimization model is shown in Eq. 17.

$$\:\begin{array}{c}\left\{\begin{array}{c}\text{min}R\\\:s.t.\left\{\begin{array}{c}200\le\:A\le\:400\\\:0.3\le\:B\le\:0.5\\\:5\le\:C\le\:15\\\:15\le\:D\le\:25\end{array}\right.\end{array}\right.\end{array}$$
(17)

The optimized combination of root-cutting elements is: the cutter rotational speed 200r/min, conveying speed 0.3 m/s, cutter inclination angle 10°, cutter overlap 20 mm; the model predicted root-cutting power is 51.19 W.

As can be seen from Fig. 9, the cutter rotational speed A has a larger slope with the response surface of other factors, i.e., the cutter rotational speed A has the greatest influence on the root cutting power R, the conveying speed B is the second largest, while the cutter inclination C and cutter overlap D have a smoother influence on the root cutting power R, i.e., C and D have the smallest influence on the root cutting power among the interacting factors.

The maximum root cutting power is the largest at 100 r/min, which is 31.147 N. The decreasing trend of the maximum root cutting power is the most obvious between the rotational speeds of the cutter of 100 r/min and 200 r/min, and the decreasing magnitude of the maximum root cutting power is smaller after the rotational speed of the cutter reaches 400 r/min. The internal energy of root cutting is positively correlated with the rotational speed of root cutting, and the larger the rotational speed of the cutter, the higher the energy consumption of root cutting. With the increase of walking speed, the maximum root cutting force increases, and at 100 r/min, the maximum root cutting force is the smallest, which is 12.449 N. With the rotational speed of the cutter between 0.2–0.4 m/s, the maximum root cutting force has the most obvious upward tendency, and after the walking speed reaches 0.5 m/s, the maximum root cutting force is caused by the effect of the impact, and its change is small. The maximum root-cutting force and internal energy of root-cutting decreased and then increased with the increase of walking speed, and the maximum root-cutting force and internal energy of root-cutting decreased and then increased with the increase of the tilt angle of the cutter and the amount of overlap of the cutter, and the maximum root-cutting force was the smallest when the amount of overlap of the cutter was 20 mm, which was the optimal amount of overlap of the root-cutting.

Conclusion

In this paper, according to the root cutting process of heading vegetables, a root cutting device for heading vegetables was designed by analyzing the kinematics and force between the root cutting of heading vegetables and the cutter, and simulation analysis and orthogonal experiments were carried out, and the main conclusions were as follows:

  1. (1)

    According to the kinematics and force analysis of the root cutting process, the maximum forward speed of the harvester was obtained when the non-leakage cutting condition was the minimum working speed of the cutter; and the combination of the cutter was determined to be the combination of the light-edged disc cutter and the serrated disc cutter.

  2. (2)

    Through ANSYS modal analysis, the reasonableness of the design of the cutter knife and cutter disk was verified, and LS-DYNA was used to carry out single-factor experiments on the four factors of walking speed, rotational speed of the cutter knife, inclination angle of the cutter knife, and overlap amount of the cutter knife in the process of root cutting, with the maximal root-cutting force and root-cutting energy as the indexes, and the influences of the four factors on the maximal root-cutting force and root-cutting internal energy were obtained.

  3. (3)

    A four-factor, three-level quadratic rotation orthogonal test was carried out on the four factors of cutter rotational speed, conveying speed, cutter inclination angle and cutter overlap amount, and through the significance analysis of the model, the factors affecting the root cutting power in the following order: cutter rotational speed A > conveying speed B > cutting cutter inclination angle C > cutting cutter overlap amount D. When the rotational speed of the cutter was 200r/min, conveying speed was 0.3 m/s, the inclination angle of the cutter was 10°, and the cutter overlap amount was 20 mm, the root cutting power of the root cutting device is lowest.

  4. (4)

    As this paper only researches on the cutting heel device, not promoted to the field test of the whole machine, the subsequent development and promotion of the whole machine can solve the problem of mechanized harvesting of nodular vegetables, and further improve the harvesting efficiency to liberate the labor force, in the future, we will continue to improve the modified device and develop the intelligent harvesting autonomous navigation system, which will be applied to the harvester, avoiding the failure of the rows due to the blindness of the operator’s field of vision, resulting in the failure of the cutting of roots Failure phenomenon.